Separation Techniques

Biography

Biography: Karel Procházka

Abstract

The general principles of chromatographic separation of mixtures of linear and branched polymers were studied by Monte Carlo simulations. The study focuses on the conditions relevant for experimental chromatography, i.e., on the partitioning of fairly dilute solutions between bulk solution and mildly sterically confined pores. The polymer chains were modeled as self-avoiding walks either in a good or in theta solvent. The partition coefficient of branched chains (K_B) is higher than the partition coefficient of linear chains (K_L) in pores with non-attractive walls and the difference between both coefficients evaluated in mixtures of polymers with different chain architectures exceeds that based on values for single chain systems. However, it decreases with increasing adsorption strength of chains on pore walls, i.e., with increasing attractive interaction between pore walls and polymer structural units, |ε|. For ε ca. -0.18, the partition coefficients equal (K_L = K_B) and later their sequence inverts. Evaluation of concentration profiles and structural characteristics of the chains at different distances from the wall reveals that more deformable linear polymers preferentially accumulate close to the attractive walls and orient parallel with the wall. The study shows that the stationary phase in neat size exclusion chromatography has to be optimized from the physico-chemical point of view, because the separation efficiency can be significantly deteriorated by its improper choice. However, the combination of size exclusion and interaction chromatography can be used for analysis of complex systems (e.g., mixtures of polymers and copolymers) when it is desirable to suppress the separation with respect to some molecular characteristics and separate the components differing in other characteristics. E.g., the combined chromatographic technique can be employed for the analysis of block copolymers because the separation can be tuned and focused on structural features of one of blocks only.

Image

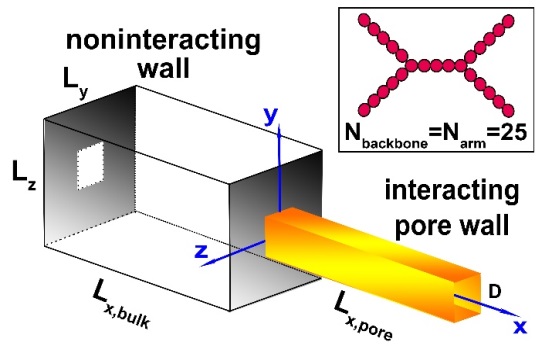
Figure 1: Schematic illustration of the simulation box. Insert shows one of studied branched structures.

Publications

1. Wang X, Lísal M, Procházka K, Limpouchová Z (2016) A Monte Carlo study of H-shaped and linear homopolymers in good solvent. Macromolecules 49: 1093-1102.
2. Posel Z, Limpouchová Z, Šindelka K,  Lísal M, Procházka K (2014) Dissipative particle dynamics study of the pH-dependent behavior of poly(2-vynilpyridine)-block-poly(ethylene oxide) diblock copolymer in aqueous buffers. Macromolecules 47: 2503-2514.
3. Šindelka K, Limpouchová Z, Lísal M., Procházka K (2014) Dissipative particle dynamics study of electrostatic self-assembly in aqueous mixtures of copolymers containing one neutral water-soluble and one either positively or negatively charged polyelectrolyte block. Macromolecules 47: 6121-6134.

4. Lísal M, Limpouchová Z, Procházka K (2016) The self-assembly of copolymers with one hydrophobic and one polyelectrolyte block in aqueous media. Physical Chemistry Chemical Physics 18:  16127-16136.
5.  Šindelka K, Limpouchová Z, Lísal M., Procházka K (2016) The electrostatic co-assembly in non-stoichiometric mixtures of copolymers composed of one neutral and one polyelectrolyte (either positively or negatively)charged) block: A dissipative particle dynamics study. Physical Chemistry Chemical Physics 18: 16137-16147.
6. Wang X., Limpouchová Z, Procházka K (2016) Computer study of chromatographic separation of mixtures of H-shaped and linear polymers in good and theta solvents. Polymer 104: 10-21.
7. Netopilík M, Janata M, Svitáková R, Trhlíková O, Berek D, Malcova E, Limpouchová Z, Procházka K (2016) Chromatographic study of the conformational behavior of grafts copolymers with a broad distribution of grafting densities in dilute solutions in selective solvents for grafts. Journal of Liquid Chromatography and Related Technologies 39: 50-58.